CIMA F3: Capital Asset Pricing Model

The Capital Asset Pricing Model (CAPM) is a CIMA F3 subject that CIMA students often struggle to get their hands around.

Astranti’s Ultimate Guide to CIMA F3 has highlighted this as one of the harder topics in F3, it’s easy to fall into the trap of memorising the formula without understanding the underlying principle.

Alternatively, students can immediately be put on the backfoot with faced with a new formula with a funky Greek symbol.

In this blog post I aim to explain the principle and logic behind the CAPM formula and how to apply it on exam day.

Let’s start with the formula itself before we take a step back and actually think about what it’s trying to achieve.

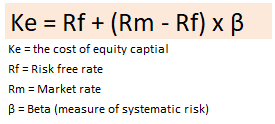
What is the Beta β Factor?

Before we get into the formula and some calculations, let’s focus on the Greek symbol for Beta (β).

The Beta factor is simply a measure of the systematic risk of a security or equity relative to the market portfolio.

Simply put, it’s a measure of volatility or risk.

The starting point would be a Beta of 1.0 – this is the benchmark for listed companies – it indicates the average risk in the market.

Let’s say Company A has a Beta of 1.0 at the start of the fiscal year, six months later the Beta drops to 0.65, this would indicate the risk profile has decreased significantly. It’s a less risky proposition to invest in but the returns would also reflect that and be at the lower end.

Had the Beta increased to 1.65, this would mean the risk profile has increased and investors would want to see a higher return for their investment due to the volatility.

Here are some real life examples of Beta factors as at 4th January 2023

Alphabet INC (GOOG) 1.06

Amazon (AMZN) 1.19

Apple (AAPL) 1.22

Netflix (NFLX) 1.28

Tesla (TSLA) 1.91

I’ve put together some household names, with the relatively safe havens of Google, Apple and Amazon with their Beta factors just over the market average.

While you can see that Tesla, given their current climate with Elon Musk’s antics and the squeeze on the automotive industry, has a high beta factor of 1.91.

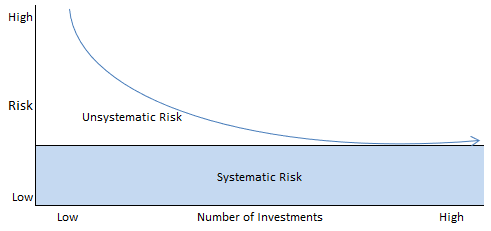
Systematic Risk and Unsystematic Risk

Now we understand our little Greek friend β, let’s focus on the types of risk involved when thinking about the Capital Asset Pricing Model.

Whenever a person wants to invest in a stock or a company invests in a project, there is always risk attached. The return on the investment will ultimately be higher or lower than expected, unless you invest in gilts or government bonds, risk is unavoidable.

There are two types of risk when investing.

Systematic risk and unsystematic risk, the Beta factor is a measure of the systematic risk of an investment.

Another way to think about systematic risk is by calling it Market Risk – it’s unavoidable, you cannot take any measures to avoid systematic risk completely. It will always be there.

Unsystematic risk, however, can be reduced or even eliminated entirely by diversification as it relates to the risk of individual company or sector. So by having a broad portfolio of investments, you will be reducing the unsystematic risk of a single investment.

The below chart nicely pulls together both types of risk and how to eliminate the unsystematic risk, while illustrating the fact systematic risk will always be there regardless on how much you diversify.

The Market Risk Premium 

The final piece of the puzzle is the Market Risk Premium of the formula, highlighted in yellow below, this is the difference between the risk free rate and the market rate over the same period.

Effectively giving you the Market Risk premium, which is the excess of market returns over those connected with so called risk free investing in Government bonds.

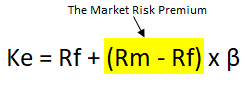
The Capital Asset Pricing model boils down to the principle that returns made in shares in the market should be higher than the returns made on risk free investments.

For example, if the risk free returns on Government bonds is 5% and the market returns are 8.5%, then the Market Risk Premium is 3.5%. Simple really.

Now we understand the various elements of CAPM, we can piece them together with some exam question examples.

CIMA F3 CAPM Exam Question Examples

1. The risk free rate of return is 6%, while the average market return is 11% and company X’s beta is 1.45. What is the cost of equity?

Given the risk involved, investors would be looking for a return of 13.25%. To give context, we can use the same example and change the Beta to 0.5 and show the impact on the cost of equity.

Ke = Rf + (Rm – Rf) x β

? = 6% + (11% – 6%) x 0.50

? = 6% + 5% x 0.50

? = 6% + 2.5%

Therefore, cost of equity is 8.5%.

As the risk profile of the investment is lowered, so are the expected returns.

Alternatively, you might be faced with a question that asks you to derive the Beta factor from the equation.

The formula and logic remains the same, you’ll just need to re-arrange the formula so the Beta becomes the calculated number and not the cost of equity.

2. Company Y plc. is giving a return of 17%, while the stock exchange as a whole is giving a 14% return and the risk free return on government issued bonds is 6%. What is the Beta (β) of Company Y plc?

Ke = Rf + (Rm – Rf) x β

17% = 6% + (14% – 6%) x ?

STEP 1: deduct the 6% from both sides of equation

11% = (14% – 6%) x ?

STEP 2: calculate the market risk premium

11% = 8% x ?

STEP 3: flip the ? to front of equation by diving both sides by 8%

? = 11%/8%

STEP 4: calculate the Beta by dividing 11% by 8%

Beta (β) = 1.375 (or 1.38)

The Capital Asset Pricing Model Summary

The Capital Asset Pricing Model (CAPM) shows the minimum required return (cost of equity) on a security, investment or project and it’s dependent on risk.

Risk can be defined as Unsystematic risk that can be negated by diversification, or Systematic Risk, but this is always inherent in the market or investment and is measured by the Beta (β) factor.

Risk averse companies or investors will seek investments or projects with a lower Beta factor. While those chasing higher gains and rewards will look towards investments with a higher Beta .

Once you’ve grasped the concept of CAPM, you should be practicing multiple choice questions to sharpen your skills for exam day!

Good Luck!